What is 0.77777 repeating as a fraction?
We’ll use a simple algebraic method to solve this.
First, let x equal our repeating decimal:
x = 0.77777
Now, multiply both sides of the equation by 10:
10x = 7.77777
Next, subtract the first equation (x = 0.77777) from the second equation (10x = 7.77777):
10x – x = 7.77777 – 0.77777
This simplifies to:
9x = 7
Dividing both sides of the equation by 9, we find that x = 7/9.
Therefore, the fraction for the recurring decimal 0.77777 is 7/9.
Let’s dive a little deeper into why this method works:
The key is understanding that multiplying a repeating decimal by a power of 10 shifts the decimal point to the right, aligning the repeating part. In our case, multiplying by 10 shifted the decimal one place to the right. Subtracting the original equation eliminates the repeating part, leaving us with a simple equation we can solve.
This technique works because we’re essentially subtracting the infinite repeating part from itself, leaving behind a finite value. The result is a fraction where the numerator represents the repeating block of digits and the denominator is a power of 10 minus 1, reflecting the number of repeating digits.
What is 0.7 when changed into fraction?
Here’s how you do it:
0.7 is the same as seven tenths.
* Think of it as saying “seven out of ten”.
* So, the fraction form of 0.7 is 7/10.
Let me give you a little more insight. Decimals are basically fractions where the denominator is a power of ten (like 10, 100, 1000, and so on). The number of digits after the decimal point tells you the power of ten.
* 0.7 has one digit after the decimal point, meaning it’s like 7/10.
0.07 has two digits, meaning it’s like 7/100.
* 0.007 has three digits, meaning it’s like 7/1000.
You see the pattern? You can easily convert any decimal to a fraction by following this simple rule!
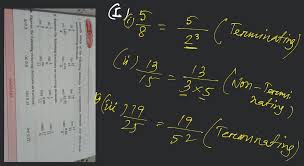
Is 0.7 repeating terminating?
0.7 is not a repeating decimal, it’s a terminating decimal. This means it has a definite ending point and doesn’t go on forever. It’s just like having a whole number, but with a decimal point.
Think of it this way: 0.7 is the same as 7/10, which is a simple fraction. You can easily write 0.7 as a fraction with a denominator that’s a power of 10 (10, 100, 1000, etc.) That’s a key characteristic of terminating decimals.
Now, let’s talk about repeating decimals. A repeating decimal has a pattern of digits that repeats endlessly. For example, 1/3 is a repeating decimal because it’s written as 0.33333… with the ‘3’ repeating forever.
Here’s the key takeaway: terminating decimals have a defined endpoint, while repeating decimals go on infinitely with a repeating pattern. Since 0.7 has a clear endpoint, it’s considered a terminating decimal.
What is 0.7 as a fraction in words?
This means that the fraction is already in its simplest form because there isn’t a common number that we can divide both 7 and 10 by.
But let’s look at this a little deeper, because it can be helpful to understand the concept of simplest form and reducing fractions.
When we talk about fractions, we’re essentially dividing one number (the numerator) by another (the denominator). In the case of 7/10, we’re dividing 7 by 10. The simplest form of a fraction means that we’ve made the numerator and denominator as small as possible while still representing the same value.
Think of it like simplifying a recipe. If you have a recipe that calls for 2 cups of flour and 1 cup of sugar, you can simplify the recipe by dividing both ingredients by 2. This results in 1 cup of flour and 1/2 cup of sugar, but it still yields the same outcome.
In the same way, we can simplify fractions by finding a common factor (a number that divides into both the numerator and denominator) and dividing both by that factor.
Let’s take the fraction 4/8 as an example. We know that 4 and 8 are both divisible by 4. So, we can divide both the numerator and denominator by 4 to get 1/2. This is the simplest form of 4/8.
But remember, in the case of 7/10, the numerator and denominator don’t have a common factor other than 1, so it’s already in its simplest form.
What fraction is 0.7 recurring?
You’re right, 0.7 is equivalent to 7/9. But let’s explore why that’s the case!
Imagine you have the decimal 0.7. Since the 7 repeats forever, we can write it as:
0.777777…
Now, let’s multiply both sides of this equation by 10:
10 * 0.777777… = 7.777777…
Next, we subtract the original equation (0.777777…) from this new equation. Notice how the repeating decimals neatly cancel out:
“`
7.777777…
– 0.777777…
————–
7.000000…
“`
This leaves us with:
7.0 = 9 * 0.777777…
Since 0.777777… is our original repeating decimal, we can rewrite it as 0.7. So, we have:
7.0 = 9 * 0.7
Finally, dividing both sides by 9 gives us:
7/9 = 0.7
Therefore, 0.7 repeating is equivalent to the fraction 7/9.
Now, let’s apply this logic to 1.2 repeating. We can write it as:
1.222222…
Following the same steps, we multiply by 10:
10 * 1.222222… = 12.222222…
Subtracting the original equation:
“`
12.222222…
– 1.222222…
————–
11.000000…
“`
We are left with:
11.0 = 9 * 1.222222…
Replacing 1.222222… with 1.2:
11.0 = 9 * 1.2
Dividing both sides by 9:
11/9 = 1.2
Therefore, 1.2 repeating is equivalent to the fraction 11/9.
You can use this same method to convert any repeating decimal into a fraction. Just remember to multiply by a power of 10 that shifts the decimal to align the repeating part, then subtract the original equation to eliminate the repeating digits. The result will be a fraction representing your repeating decimal!
Why is 0.7 repeating rational?
The key to understanding this is that any number that can be written as a fraction is a rational number. A rational number is simply a number that can be expressed as the ratio of two integers, with the denominator not being zero. For example, 1/2 , 3/4, or even 5/1 are all rational numbers.
Now, let’s see how we can express 0.7 repeating as a fraction.
Let’s say x = 0.7777…
Multiply both sides of this equation by 10:
10x = 7.7777…
Subtracting the first equation from the second equation, we get:
10x – x = 7.7777… – 0.7777…
This simplifies to 9x = 7
Dividing both sides by 9, we get x = 7/9.
Therefore, we have successfully expressed 0.7 repeating as the fraction 7/9. This proves that 0.7 repeating is a rational number because it can be represented as a ratio of two integers.
Is 0.7 the same as 7?
Let’s break it down:
7 is a whole number, representing seven complete units.
0.7 is a decimal, representing seven tenths of a whole. Think of it like cutting a pie into ten slices. 0.7 represents seven of those slices, which is less than the whole pie.
Imagine you have seven apples, that’s 7. Now, imagine you have seven out of ten apple slices, that’s 0.7. You have less than a whole apple in the second example.
Understanding the difference between whole numbers and decimals is crucial when working with numbers. It’s like recognizing the difference between a dollar and a dime – one is worth more than the other. Knowing this will help you perform calculations correctly and avoid mistakes.
What is .7 as a fraction?
Let’s break this down a bit more. Decimals represent parts of a whole number. The decimal point separates the whole number from the fractional part. In 0.7, the “7” is in the tenths place. This means it represents seven out of ten equal parts of the whole.
Think of it like a pizza. If you cut the pizza into ten slices, and you take seven of those slices, you’ve taken 7/10 of the pizza! And that’s exactly what 0.7 represents.
See more here: What Is 0.7 When Changed Into Fraction? | What Is 0.7 Recurring As A Fraction
What is 0.7 repeating as a fraction?
First, we’ll set up an equation where x equals 0.7 repeating.
x = 0.777777…
Next, we’ll multiply both sides of the equation by 10:
10x = 7.777777…
Now, we’ll subtract the first equation (x = 0.777777…) from the second equation (10x = 7.777777…). This will cancel out the repeating decimals:
10x – x = 7.777777… – 0.777777…
9x = 7
Finally, we’ll solve for x by dividing both sides of the equation by 9:
x = 7/9
So, 0.7 repeating is equivalent to the fraction 7/9.
Understanding the Process
The key to converting repeating decimals to fractions is recognizing the pattern of repetition. By multiplying the original decimal by a power of 10 (in this case, 10), we shift the decimal point one place to the right. This allows us to subtract the original decimal, effectively eliminating the repeating portion.
The resulting equation (9x = 7) represents a simple equation with a whole number on one side and a fraction on the other. Solving for *x* gives us the fractional equivalent of the repeating decimal.
This technique can be applied to any repeating decimal. The trick is to identify the repeating block and use the appropriate power of 10 to align the decimals for subtraction.
What does 0.7 repeating mean?
Let’s break down how to convert this repeating decimal into a fraction. Here’s the formula:
N/(10^n – 1)
N represents the repeating part of the decimal (in our case, 7).
n is the number of digits in the repeating block (in this case, 1 because only the 7 repeats).
So, plugging in our numbers, we get 7/(10^1 – 1). Simplifying, we get 7/(10 – 1), which equals 7/9.
That’s it! 0.7 repeating is the same as the fraction 7/9.
It’s kind of like saying that we have 7 pieces of a pie that’s been divided into 9 equal pieces.
What is 7/9 repeating as a fraction?
The answer is quite simple: 7/9 is already a fraction! It’s just that it’s expressed in its simplest form. When you divide 7 by 9, you get a repeating decimal, 0.777777….
But there’s a way to express any repeating decimal as a fraction! Let’s explore how:
Understanding Repeating Decimals
Repeating decimals occur when a number’s decimal representation continues infinitely with a pattern of repeating digits. The repeating part is often indicated with a bar over the digits, such as 0.333… (written as 0.3̅), or 0.142857142857… (written as 0.142857̅).
Converting Repeating Decimals to Fractions
Let’s take your example of 0.777777… (0.7̅) and see how to convert it to a fraction:
1. Set up an equation: Let *x* equal the repeating decimal:
*x* = 0.7̅
2. Multiply to shift the decimal: Multiply both sides of the equation by 10, as there’s one repeating digit:
10*x* = 7.7̅
3. Subtract the original equation: Subtract the original equation (*x* = 0.7̅) from the new equation (10*x* = 7.7̅):
10*x* – *x* = 7.7̅ – 0.7̅
9*x* = 7
4. Solve for x: Divide both sides by 9:
*x* = 7/9
Therefore, the fraction representation of 0.7̅ is 7/9. This method works for any repeating decimal!
How to convert a repeating decimal number to a fraction?
The Formula:
We’ll use a simple formula to convert any repeating decimal to a fraction. Here’s the breakdown:
N: The non-repeating part of the decimal number.
R: The repeating part of the decimal number.
P: The number of digits in the repeating part.
Example: Let’s convert 0.7 (repeating) into a fraction.
1. Identify N, R, and P:
N = 0 (There is no non-repeating part)
R = 7 (The repeating digit)
P = 1 (There’s one digit in the repeating part)
2. Apply the Formula:
Fraction = (N x 10^P + R) / (10^P – 1)
3. Substitute and Calculate:
Fraction = (0 x 10^1 + 7) / (10^1 – 1)
Fraction = 7 / 9
Therefore, 0.7 repeating is equivalent to 7/9 as a fraction.
Understanding the Logic:
The formula works because it cleverly manipulates the repeating decimal to create an equation that eliminates the repeating part. Let’s break it down:
Multiplying by 10^P: This shifts the decimal point to the right by P positions, making the repeating part align perfectly.
Subtracting the original decimal: This cancels out the repeating portion, leaving us with a whole number.
Dividing by (10^P – 1): This isolates the fraction, giving us the equivalent representation.
Let’s illustrate with our example:
10 x 0.7777… = 7.7777… (Multiplying by 10, we shifted the decimal)
0.7777… – 7.7777… = -7 (Subtracting the original decimal cancels the repeating portion)
-7 / 9 = 7 / -9 (We divide by (10^1 – 1) = 9)
And voila, we’ve arrived at 7/9!
This formula simplifies the process of converting repeating decimals to fractions, making it a much more manageable task.
See more new information: countrymusicstop.com
What Is 0.7 Recurring As A Fraction? A Simple Explanation
Let’s dive in!
Understanding Recurring Decimals
First off, let’s talk about what we mean by “recurring decimal”. A recurring decimal, also called a repeating decimal, is a decimal number that has a repeating pattern of digits after the decimal point. In our case, we’re dealing with 0.7 recurring. This means the “7” repeats forever: 0.7777777… and so on.
Converting Recurring Decimals to Fractions: The Steps
Here’s the breakdown of how to convert 0.7 recurring into a fraction:
1. Set up an equation: Let’s say x is equal to our recurring decimal, 0.7 recurring. So, we have:
x = 0.777777…
2. Multiply to shift the decimal: Our goal is to have the repeating part of the decimal on both sides of the equation. We multiply both sides of our equation by 10. This gives us:
10x = 7.777777…
3. Subtract the original equation: Now, we subtract the original equation (x = 0.777777…) from the equation we just got (10x = 7.777777…). Notice how the repeating “7s” cancel out:
“`
10x = 7.777777…
– x = 0.777777…
—————-
9x = 7
“`
4. Solve for x: We’re left with a simple equation: 9x = 7. To isolate x, we divide both sides by 9:
x = 7/9
And there you have it! 0.7 recurring is equal to 7/9.
Let’s Summarize:
We just converted 0.7 recurring to its fractional form using these steps:
1. Represent the decimal as ‘x’.
2. Multiply both sides of the equation by 10 to shift the decimal.
3. Subtract the original equation from the multiplied equation.
4. Solve for ‘x’ by dividing both sides by the coefficient of ‘x’.
Let’s do Another Example:
Let’s try a slightly different example. Let’s say we have 0.36 recurring. This means it’s 0.36363636…
Following the same steps:
1. x = 0.363636…
2. 100x = 36.363636… (multiplying by 100 to shift the decimal two places)
3. 100x = 36.363636…
– x = 0.363636…
——————–
99x = 36
4. x = 36/99
5. x = 4/11 (Simplifying the fraction)
So, 0.36 recurring is equal to 4/11.
FAQs
You might have some questions about recurring decimals and converting them to fractions. Here are some common ones:
1. What if the repeating block has more than one digit?
No problem! Let’s say we have 0.123123123… We follow the same principle:
1. x = 0.123123…
2. 1000x = 123.123123… (multiplying by 1000 to shift the decimal three places)
3. 1000x = 123.123123…
– x = 0.123123…
——————–
999x = 123
4. x = 123/999
5. x = 41/333 (Simplifying the fraction)
2. What if the repeating part doesn’t start immediately after the decimal point?
Let’s say we have 0.567777…
1. x = 0.567777…
2. 10x = 5.677777… (multiplying by 10 to shift the decimal one place)
3. 100x = 56.777777… (multiplying by 100 to shift the decimal two places)
4. 100x = 56.777777…
– 10x = 5.677777…
——————–
90x = 51
5. x = 51/90
6. x = 17/30 (Simplifying the fraction)
3. How do I know what to multiply by?
You multiply by the power of 10 that corresponds to the number of digits in the repeating block. If you have one repeating digit, multiply by 10. If you have two repeating digits, multiply by 100, and so on.
So, for 0.11111…, you multiply by 10. For 0.323232…, you multiply by 100.
4. Is there a shortcut for converting recurring decimals to fractions?
There isn’t a super quick shortcut, but there’s a way to simplify the process for certain decimals:
For decimals with a single digit repeating: Write the repeating digit as the numerator and the number of 9s in the denominator equal to the number of repeating digits. For example, 0.333… is 3/9, which simplifies to 1/3.
For decimals with a block of repeating digits: Write the repeating block as the numerator and a string of 9s in the denominator equal to the number of repeating digits. For example, 0.123123… is 123/999, which can be simplified further.
Remember, these shortcuts are just a helpful way to visualize the process, but the method we explained earlier is the most reliable for any recurring decimal.
0.7 Repeating as a Fraction – Calculation Calculator
What is 0.7 repeating as a Fraction? Here’s how to convert 0.7 repeating as a Fraction using the formula, step by step instructions are given inside. Calculation Calculator
Repeating Decimal to Fraction Conversion Calculator
You can use this repeating decimal to fraction conversion calculator to revert a repeating decimal to its original fraction form. Simply input the repeating part of the decimal (the Good Calculators
What is 0.7 repeating as a fraction? – Number Maniacs
Learn how to convert 0.7 repeating to a fraction using a simple formula. The answer is 7/9 in the simplest form. Number Maniacs
Converting repeating decimals to fractions (part 2 of 2) – Khan
Repeated decimals can be converted into fractions by shifting the decimal to the right and subtracting the decimals. To do this, multiply the number by 10 to the second power, Khan Academy
Converting repeating decimals to fractions (part 1 of 2) – Khan
Learn how to convert 0.7 repeating to 7/9 and other repeating decimals to fractions with 9 as the denominator. Watch a video, see examples and practice problems, and read Khan Academy
How To Convert Recurring Decimals To Fractions (Step
To convert a recurring decimal to a fraction, start by writing out the equation where (the fraction we are trying to find) is equal to the given number. Use a few repeats of the recurring decimal here. For Practice Aptitude Tests
Decimal Repeating as a Fraction Calculator – Number Maniacs
The Decimal Repeating as a Fraction Calculator can explain and convert repeating decimal numbers to fractions for you. Number Maniacs
Convert a repeating decimal to a fraction – Wolfram|Alpha
The widget converts your repeating decimal to fraction form. Be sure to enter the repeating part of your decimal twice. Wolfram|Alpha
Writing repeating decimals as fractions review – Khan
Subtract the two equations: 9 x = 7. Solve for x : x = 7 9. Remember from the first step that x is equal to our repeating decimal, so: 0. 7 ― = 7 9. Want to learn more about writing Khan Academy
How To Convert Recurring Decimals To Fractions (Proportions Part 6/6) #18
Write As A Fraction 0.7 Repeating
0.7 As A Fraction (Simplified Form)
The Maths Prof: Changing Recurring Decimals Into Fractions
Convert 0.7 To A Fraction . Convert Repeating Decimal To A Fraction, Recurring Decimals 0,7
Link to this article: what is 0.7 recurring as a fraction.
See more articles in the same category here: blog https://countrymusicstop.com/wiki
