Minimum Angle Of Deviation Prism Experiment | How To Find Angle Of Minimum Deviation In Prism?

The angle of minimum deviation is the smallest angle of deviation a prism can produce. This occurs when the angle of incidence and angle of emergence are equal. The angle of minimum deviation is determined by the prism’s refracting angle and the material’s refractive index.

Let’s break down how this works.

Imagine a ray of light entering a prism. As the light passes through the prism, it bends, or refracts. The amount of bending depends on the angle at which the light enters the prism, the refracting angle of the prism (the angle between the two faces of the prism), and the refractive index of the prism material.

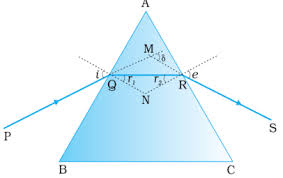
Now, as you gradually increase the angle of incidence, the angle of deviation first decreases, reaches a minimum value, and then starts increasing again. This minimum angle of deviation is the angle of minimum deviation. The key takeaway is that at the angle of minimum deviation, the incident ray and emergent ray are symmetric with respect to the base of the prism.

So, how do you find the angle of minimum deviation? Here’s a breakdown of the steps:

1. Shine a light beam through the prism. Use a narrow beam of light, like a laser pointer.
2. Adjust the angle of incidence. This is the angle between the incident ray and the normal to the surface of the prism. You can do this by rotating the prism or moving the light source.
3. Observe the angle of deviation. This is the angle between the incident ray and the emergent ray.
4. Find the angle of incidence where the angle of deviation is the smallest. This is the angle of minimum deviation.

You can also calculate the angle of minimum deviation using a formula:

δm = 2i – A

Where:

δm is the angle of minimum deviation
i is the angle of incidence (and emergence) at minimum deviation
A is the refracting angle of the prism

Keep in mind that this formula applies when the prism is made of a material with a refractive index greater than 1.

The angle of minimum deviation is a useful concept in optics. It can be used to determine the refractive index of a prism material. It also plays an important role in understanding the behavior of light as it passes through prisms and other optical elements.

Let’s talk about the minimum incident angle of a prism. You might be wondering what that means and why it’s important.

It turns out, there’s no single minimum incident angle that applies to all prisms. The minimum incident angle depends on a few things:

The type of prism: Different prism shapes (like triangular prisms, rectangular prisms) will have different angles and refractive indices.
The material of the prism: The material the prism is made of (like glass, plastic, or even water) determines how much light bends as it passes through.
The desired outcome: Are you trying to get a specific color of light out of the prism? Or are you interested in achieving a specific angle of deviation?

The minimum incident angle is the smallest angle at which light needs to enter the prism to undergo a specific kind of interaction, like internal reflection or refraction. To understand this better, imagine shining a light beam at a prism. As the light enters the prism, it bends. If the light hits the prism at a very shallow angle (a small incident angle), it might pass straight through with little bending. But as you increase the angle of incidence, the light bends more and more.

There’s a certain angle where the light will be bent so much that it gets reflected back inside the prism. This is called total internal reflection, and it’s a key principle in how prisms work. To achieve total internal reflection, you need to exceed a certain critical angle, which depends on the prism’s material.

So, the minimum incident angle for a prism is the angle that’s just large enough to achieve the desired outcome, whether that’s a specific angle of deviation or total internal reflection.

Keep in mind that the angle of incidence is the angle between the incoming light ray and a line perpendicular to the surface of the prism. It’s crucial for understanding how light interacts with prisms.

We measured the angle of minimum deviation in a spectrometer experiment and found it to be 48.6 degrees.

This measurement is important because it helps us understand the properties of the prism. The angle of minimum deviation is the smallest angle at which a ray of light can pass through the prism and emerge from the other side. This angle is dependent on the refractive index of the prism and the angle of the prism itself.

When a beam of white light is passed through a prism, it gets dispersed into its constituent colors because each color has a different refractive index. This phenomenon is called dispersion. The angle of minimum deviation is also related to the dispersion of light by the prism. The smaller the angle of minimum deviation, the more dispersed the light will be.

To find the angle of minimum deviation, we use a spectrometer. A spectrometer is an instrument that uses a prism to separate light into its constituent colors. The spectrometer has a collimator, a prism, and a telescope. The collimator makes the incident light parallel, the prism disperses the light, and the telescope focuses the light onto a screen.

To measure the angle of minimum deviation, we first adjust the prism so that the light beam passes through it symmetrically. We then rotate the telescope until the image of the slit appears in the center of the field of view. The angle between the direction of the incident light and the direction of the emergent light is called the angle of deviation. We then rotate the prism slightly and measure the angle of deviation again. We repeat this process for different angles of incidence.

The angle of minimum deviation occurs when the angle of deviation is at its minimum value. This happens when the angle of incidence is equal to the angle of emergence. At this point, the angle of deviation is independent of the angle of incidence.

Knowing the angle of minimum deviation allows us to calculate the refractive index of the prism. This is a crucial piece of information for understanding the optical properties of materials.

We can determine the angle of minimum deviation experimentally by measuring a few key angles. First, we need to know the apex angle (α) of the prism, which is the angle between the two sides of the prism. Next, we need to measure the angle of incidence (θi1) on the first surface of the prism. Finally, we need to measure the angle of minimum deviation (δ) for the refraction at the second surface.

With these measurements, we can calculate the refractive index (n) of the prism material. The angle of minimum deviation occurs when the angle of incidence and the angle of emergence are equal. At this point, the path of the light ray through the prism is symmetrical, and the angle of deviation is at its minimum.

Let’s break down the process of determining the angle of minimum deviation:

1. Set up the Experiment: We start by setting up a prism on a prism table with a source of light, like a sodium lamp, placed in front of it. The prism table is a platform with a circular scale and a vernier scale that helps measure angles accurately.

2. Finding the Angle of Minimum Deviation: We shine the light beam through the prism and rotate the prism until the emergent ray is at its minimum deviation. We then measure the angle of deviation using the vernier scale on the prism table.

3. Measuring the Apex Angle: The apex angle of the prism can be measured using a spectrometer.

4. Calculating the Refractive Index: We can then use the following formula to calculate the refractive index (n) of the prism material:

n = sin((α + δ)/2) / sin(α/2)

By following these steps, we can accurately determine the angle of minimum deviation and calculate the refractive index of the prism material. This experiment is a fundamental concept in optics and helps us understand the behavior of light as it passes through different materials.

Let’s dive into the fascinating world of prisms and understand what factors influence the minimum deviation!

The minimum deviation of a prism is the smallest angle by which a light ray is deflected after passing through it. This minimum deviation occurs when the angle of incidence and the angle of emergence are equal. There are several factors that play a key role in determining this minimum deviation, and we’ll explore each one in detail:

Angle of Incidence: This is the angle at which the light ray strikes the prism’s first surface. Think of it as the “entry angle” for the light. Changing this angle directly affects the path of the light ray within the prism and consequently the minimum deviation.
Wavelength of Light: Different colors of light, or different wavelengths, bend at different angles as they pass through a prism. This is why we see a beautiful rainbow when white light passes through a prism! Each color has its own specific minimum deviation.
Material of the Prism: The material of the prism, like glass or plastic, influences how much light bends as it passes through. The refractive index of the material is the key factor here – a higher refractive index means the light bends more, resulting in a greater minimum deviation.
Angle of the Prism: This refers to the angle between the two refracting surfaces of the prism. A larger angle means more bending of the light, leading to a larger minimum deviation.

Let’s get a little more technical! The relationship between the refractive index of the prism material (μ), the angle of the prism (A), and the minimum deviation (δm) is expressed by a simple equation:

μ = sin((A + δm)/2) / sin(A/2)

This equation tells us that the refractive index of the prism material is directly proportional to the sine of half the sum of the angle of the prism and the minimum deviation, all divided by the sine of half the angle of the prism. So, you can see how all these factors are interconnected!

Understanding the factors that affect the minimum deviation is crucial in various applications, such as in spectrometers used to analyze light and telescopes to focus light. By carefully controlling these factors, scientists and engineers can manipulate the path of light with precision.

Let me know if you want to dive into a specific factor or application in more detail – I’m always here to help you unravel the mysteries of light and prisms!

We know that the angle of minimum deviation of a prism is the smallest angle of deviation that a ray of light undergoes when it passes through the prism. The effective angle of a prism is the angle between the two refracting surfaces of the prism.

For the angle of minimum deviation to be equal to the effective angle, the prism must be made of a material whose refractive index μ is between √2 and 2. This means that the light bends more when it enters the prism than when it leaves the prism.

Let’s break this down.

What exactly is the relationship between the angle of minimum deviation and the refractive index of the prism?

The angle of minimum deviation is related to the refractive index of the prism by the following formula:

“`
δm = 2i – A
“`

where:

δm is the angle of minimum deviation
i is the angle of incidence
A is the effective angle of the prism

This formula tells us that the angle of minimum deviation is directly proportional to the refractive index of the prism. This means that if the refractive index increases, the angle of minimum deviation will also increase.

Let’s now look at the case when δm = A.

In this case, the formula becomes:

“`
A = 2i – A
“`

Solving for i, we get:

“`
i = A
“`

This tells us that the angle of incidence is equal to the effective angle of the prism. Now, applying Snell’s law at the first surface of the prism:

“`
sin i / sin r = μ
“`

where r is the angle of refraction.

Since i = A, we get:

“`
sin A / sin r = μ
“`

Now, applying Snell’s law at the second surface of the prism:

“`
sin r / sin (A – r) = μ
“`

Solving for r, we get:

“`
r = A/2
“`

Substituting this value of r in the equation above, we get:

“`
sin A / sin (A/2) = μ
“`

Simplifying the above equation, we get:

“`
2cos(A/2) = μ
“`

Since cos(A/2) is always less than or equal to 1, we get:

“`
μ ≤ 2
“`

Similarly, since cos(A/2) is always greater than or equal to 0, we get:

“`
μ ≥ √2
“`

Therefore, the refractive index of the prism must be between √2 and 2 for the angle of minimum deviation to be equal to the effective angle.

We can find the minimum incident angle, also known as the critical angle, by using Snell’s Law. This law describes the relationship between the angles of incidence and refraction when light passes from one medium to another. It states that the ratio of the sine of the angle of incidence to the sine of the angle of refraction is a constant, known as the index of refraction.

Let’s break down how this works. When light travels from a denser medium, such as water, to a less dense medium, like air, it bends away from the normal. The normal is a line perpendicular to the surface at the point where the light ray hits it. As the angle of incidence increases, the angle of refraction also increases. At a specific angle, called the critical angle, the refracted ray will be bent at 90 degrees, meaning it will travel along the boundary between the two mediums.

Now, when the angle of incidence exceeds the critical angle, the light ray will no longer be refracted but instead will be reflected back into the denser medium. This phenomenon is known as total internal reflection.

To determine the critical angle, we can use Snell’s Law. Let’s assume the first medium, where the light originates, has an index of refraction n1, and the second medium has an index of refraction n2. When the angle of refraction is 90 degrees, we can write Snell’s Law as:

n1 * sin(critical angle) = n2 * sin(90°) = n2

Since sin(90°) = 1, we can simplify this equation to:

sin(critical angle) = n2 / n1

To find the critical angle, we simply take the inverse sine of both sides:

critical angle = arcsin(n2 / n1)

This equation tells us that the critical angle depends solely on the indices of refraction of the two mediums. The higher the ratio of n2 / n1, the smaller the critical angle.

Understanding the critical angle is crucial for many applications, including fiber optics, prisms, and even how our eyes work.

Let’s figure out how to find the angle of minimum deviation for a prism! We’ll do this by plotting a graph that shows the relationship between the angle of incidence and the angle of deviation.

First, we need to understand what deviation means. Deviation is the angle by which a light ray bends when it passes through a prism. Think of it like this: Imagine you’re shining a light through a window. The light will change direction slightly as it goes from the air into the glass. This change in direction is called deviation.

Now, let’s dive into the experiment.

Finding the Angle of Minimum Deviation

1. Set up the experiment: You’ll need a prism, a light source (like a laser pointer), a protractor, and a sheet of paper. Place the prism on the paper and shine the light through one of its faces.
2. Measure the angle of incidence: The angle of incidence is the angle between the incoming light ray and the normal (a line perpendicular to the surface of the prism). Adjust the light source to change the angle of incidence.
3. Measure the angle of deviation: The angle of deviation is the angle between the incoming light ray and the outgoing light ray. Measure this angle using your protractor.
4. Repeat steps 2 and 3 for different angles of incidence. You’ll want to get a good range of data.
5. Plot your data: On a graph, plot the angle of incidence on the x-axis and the angle of deviation on the y-axis.
6. Find the minimum point: You’ll notice that the graph will have a minimum point. This point represents the angle of minimum deviation. This is the smallest angle of deviation that you can get for a given prism.

Why is the angle of minimum deviation important?

The angle of minimum deviation is a useful property of a prism. It’s used in many optical instruments, like spectrometers, which are used to separate light into its different colors. The angle of minimum deviation helps us understand how light interacts with materials like prisms.

Understanding this principle helps us design and build important tools for studying light and its properties.

Let’s talk about the minimum angle of incidence in a prism. You might be wondering, what exactly is that? Well, it’s all about the way light bends when it passes through a prism.

Think of a prism like a triangular piece of glass. When light enters the prism, it gets refracted, meaning it bends. The amount of bending depends on the angle at which the light hits the prism, which is called the angle of incidence.

Now, there’s a special angle of incidence where the light bends the least amount. This is called the minimum angle of deviation. It’s kind of like finding the sweet spot where the light is deflected the least. This angle is important because it helps us determine the refractive index of the prism material, which is a measure of how much the material bends light.

The minimum angle of deviation is denoted by δmin, Dλ, or Dm. You can think of it as the “minimum deviation angle.” It’s the smallest amount of bending the light experiences as it passes through the prism.

Here’s what happens:

When the light enters the prism, it gets refracted and bends towards the normal (an imaginary line perpendicular to the surface).
As the light travels through the prism, it continues in a straight line.
When the light exits the prism, it gets refracted again and bends away from the normal.

The minimum angle of deviation occurs when the angle of incidence and the angle of emergence (the angle at which the light exits the prism) are equal. This means that the light is bent equally on both sides of the prism, resulting in the smallest possible deviation.

To understand why this happens, consider the path of a light ray as it passes through the prism. As the angle of incidence increases, the angle of deviation initially decreases. However, after a certain point, the angle of deviation starts to increase again. The minimum angle of deviation corresponds to the point where the angle of deviation is at its lowest.

So, the minimum angle of incidence is the angle at which light enters the prism to achieve this minimum angle of deviation. It’s a key concept in understanding the behavior of light as it passes through a prism.

Let’s figure out how to find the minimum angle of deviation for a prism.

To find the minimum angle of deviation, we need to create a graph. This graph shows the relationship between the angle of incidence and the angle of deviation. But first, let’s define what we mean by angle of deviation.

The angle of deviation is the angle between the incident ray and the emergent ray. In simpler terms, it’s the amount by which the light ray is bent as it passes through the prism.

Here’s how we find the minimum angle of deviation:

1. Experiment: We shine a light beam onto a prism and change the angle of incidence gradually. For each angle of incidence, we measure the corresponding angle of deviation.
2. Graph: We plot the measured angles of incidence on the x-axis and the corresponding angles of deviation on the y-axis.
3. Minimum Point: The graph will have a minimum point. This minimum point represents the smallest possible angle of deviation for that particular prism.

Why does a minimum angle of deviation exist?

Imagine shining a light beam through a prism. As you change the angle of incidence, the angle of deviation also changes. At some point, you’ll reach an angle of incidence where the light ray emerges from the prism with the least amount of deviation. This is because of the way light interacts with the prism’s surfaces. The angles at which light enters and exits the prism influence the overall deviation.

By finding the minimum point on the graph, we determine the angle of incidence that produces the smallest possible angle of deviation for that particular prism. This is a key concept in understanding how prisms work and how they can be used to manipulate light.

The minimum deviation is the smallest angle by which a prism can deviate a beam of light. It occurs when the angle of incidence and angle of emergence are equal, and the light ray travels parallel to the base of the prism.

Let’s delve deeper into what minimum deviation means:

Imagine shining a light beam through a prism. The light bends as it enters and exits the prism, resulting in a deviation from its original path. As you change the angle of incidence, the amount of deviation also changes. There’s a special angle, the angle of minimum deviation, where the deviation is at its smallest.

Here’s why minimum deviation is important:

Symmetry: At the angle of minimum deviation, the light ray travels symmetrically through the prism. This means the angle of incidence and the angle of emergence are equal.
Parallel Path: The light ray inside the prism travels parallel to the prism’s base.
Practical Applications: The concept of minimum deviation is crucial in understanding and designing optical instruments like spectrometers and prisms used in telescopes. It helps us determine the refractive index of materials and analyze the spectrum of light.

In summary, the minimum deviation is a key concept in optics. It’s the smallest possible deviation that a prism can produce, and it occurs under specific conditions of symmetry and parallel light path. It has practical implications in various optical applications.

Angle of Minimum Deviation – Lab Experiments – EMBIBE

The angle between the two refracting surfaces is called the angle of the Prism (A). The angle between the emergent ray and the incident ray is known as the angle of deviation. Let us consider a case of minimum deviation to understand our experiment better. EMBIBE

Determination of Minimum Deviation For Given

Minimum deviation is determined for a given prism by plotting graph between angle of incidence and angle of deviation. BYJU’S

Angle of minimum deviation experiment for class 12. Prism

In this video we will perform angle of minimum deviation experiment for class 12. Prism experiment. From graph we will determine angle of minimum YouTube

Topics Minimum deviation of angle in prism Masatsugu Sei

The experiment of the minimum deviation angle in prism is one of the topics in the Sophomore laboratory (SUNY-Binghamton). We use the mercury and sodium lamps as BINGWEB

To determine angle of minimum deviation for a given prism by

To determine angle of minimum deviation for a given prism by plotting a graph between angle of incidence and the angle of deviation. 1) What are the materials required for BYJU’S

Phys 1102/1220, Lab 09: Finding the angle of minimum deviation

A tutorial video for Lab 9, The Prism Spectrometer, of KPU’s Phys 1102 and Phys 1220 courses. This video covers how to correctly find the angle of minimum deviation using your… YouTube

1.6: Refraction by a Prism – Physics LibreTexts

The angle of minimum deviation \(D_{\text{min}}\) is \(2\theta_1 − \alpha\), where \(\theta_1\) is given by Equation \(\ref{eq:1.6.4}\), and this leads to the following relation between the refractive index and the Physics LibreTexts

THE PRISM SPECTROMETER – Michigan State University

When a ray of light is refracted by a prism, the angle between the incoming and outgoing rays is called the angle of deviation (β). For a given prism and a given wavelength, the web.pa.msu.edu